Research our contributions to discovery
Anomalous scalings in the diffusion of granular materials
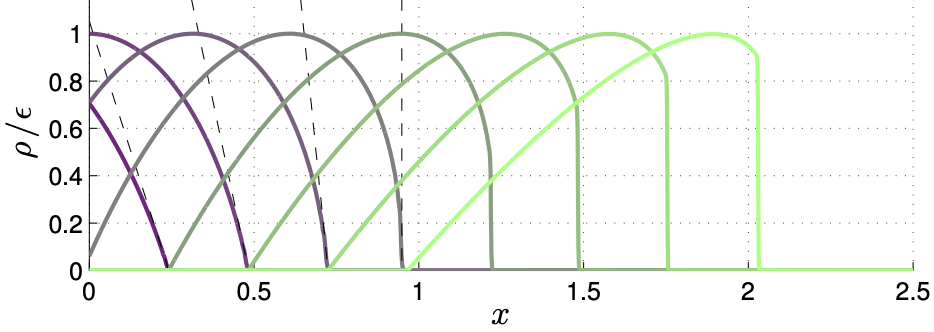
Check out our paradigm-shifting paper on the intermediate asymptotics of non-degenerate diffusion equations, contributed to the Proceedings of the National Academy of Sciences of the USA by G. I. Barenblatt himself. Research supported by the National Science Foundation.
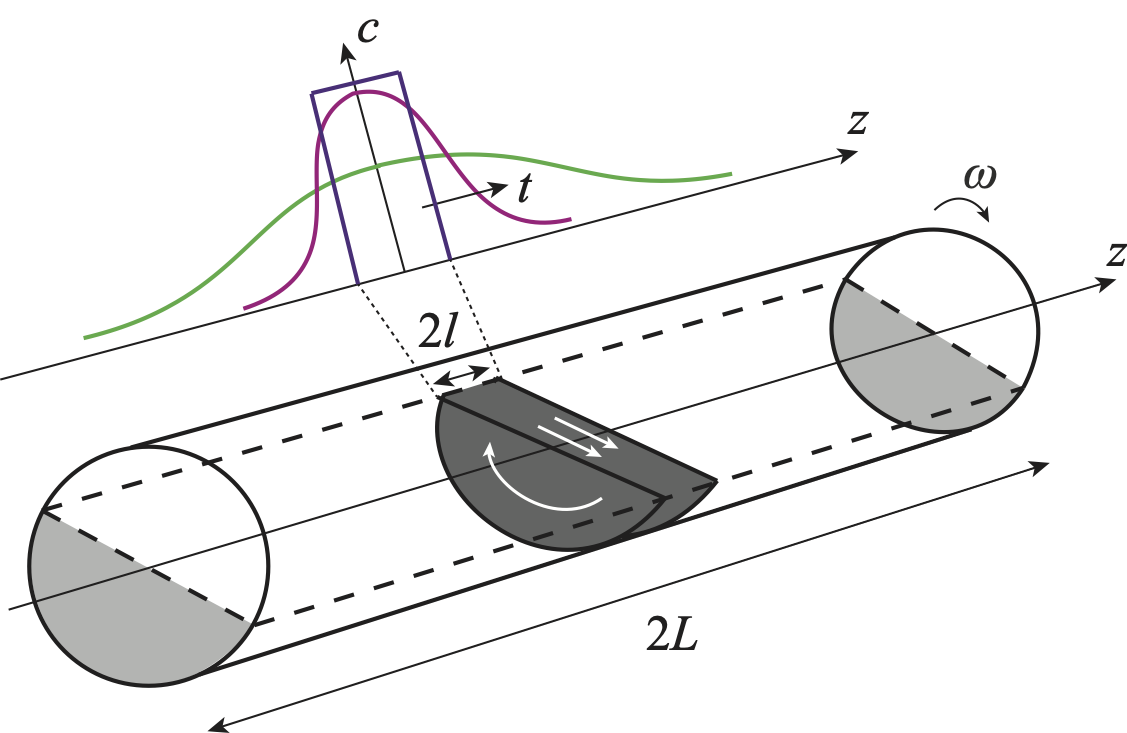
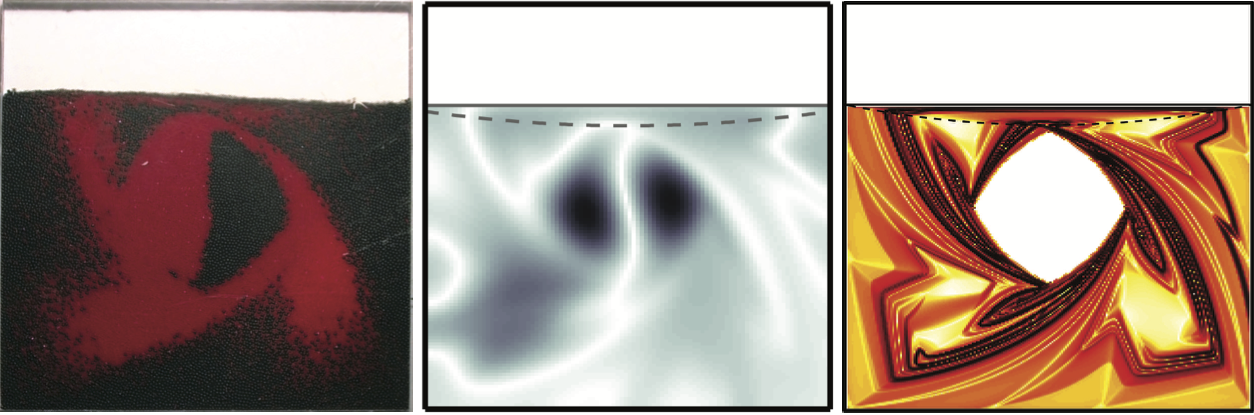
Chaotic mixing of granular materials; 3D nonlinear dynamical systems and chaos
This was the topic of the TMNT-Lab PI's PhD dissertation. He applied advanced techniques from the theory of nonlinear dynamical systems to the problem of mixing and segregation of granular materials. Research supported by the National Science Foundation.
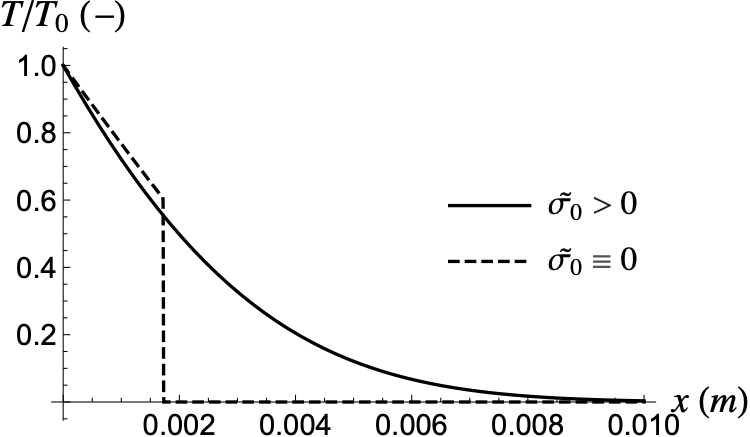
Hyperbolic heat conduction and wave propagation
Amongst several mathematical contributions to this field, mistakes were found in a paper claiming cloaking of hyperbolic heat waves. Research proudly performed on nights & weekends without expending any taxpayer funds.
Nonlinear acoustics (inviscid and thermoviscous) and shock formation
Amongst many accomplishments and contributions to this field, new weakly-nonlinear acoustic equations were derived for both lossless gasses and liquids, without suffering from the shortcomings of the approximations credited to Kuznetsov and Lighthill–Westervelt (though this attribution is somewhat incorrect).
Numerical methods for hyperbolic systems of conservation laws
This was the topic of the TMNT-Lab PI's MS research — implementing a fully-discrete non-oscillatory central scheme for hyperbolic systems of conservation on unstructured triangular meshes, including introducing a "genuinely multidimensional" non-oscillatory minimum-angle plane reconstruction (MAPR).
Wavelets and wavelet methods for signal processing and solution of PDEs
Legacy project, now archived.